M.C. Escher and Tessellations

Many of the drawings of Dutch artist Maurits Cornelis (M.C.) Escher closely connect with the mathematical concepts of infinity and contradiction. While these concepts lead to many themes, tessellations of the plane appear particularly often in Escher's work.

A tessellation (or tiling) of the plane is a construction that fills a flat surface completely with geometric shapes, usually called tiles. Escher often explored symmetric tessellations that were formed by repeatedly duplicating and rearranging only a single tile through translation, rotation and reflection. A simple example of such a tessellation is one which uses only squares — you can imagine the following pattern repeated to completely cover an arbitrarily large surface.

Or, we might have a slightly more complex construction using triangles.

From the mathematical perspective, these constructs are often quite rigid as in the above examples, but Escher discovered more complex tessellations that bring visual interest and aesthetic beauty. This complexity is found in, for example, Escher's Lizard.

The lizard's shape, while on its own not particularly symmetric, combines with copies of itself to create symmetry on a larger scale. Escher uses tessellation to highlight the artistic relationship between positive and negative space. In Lizard, there isn't a clear distinction between the subject and its background. If you view the lighter-colored lizards as the subject, then the negative space is comprised of the darker-colored lizards and vice versa. In a sense, the subject is its own negative space through the symmetric tiling.

Transformations Between the Rigid and the Organic

Escher was probably aware of the perceived rigidity of tessellations. In contrast, Escher has a few works in which he depicts the transformation from rigid geometric tilings into organic and natural imagery. Liberation shows a triangular tessellation (similar to the example above) that slowly transforms into an array of birds mid-flight.

Which are being liberated — the birds or the triangles? On the one hand, the birds seem to be breaking free from the rigid geometric form of the triangles. But on the other hand, the triangles exist in a world of mathematical perfection which has a beauty of its own.

The transformation demonstrates one technique for constructing complex tilings by making small perturbations to an existing tiling. This process is detailed at the end of the post.

Tessellations in Non-Euclidean Geometries

Escher's Circle Limit drawings — Circle Limit I is pictured at the beginning of this post and Circle Limit III is pictured above — display a different kind of tiling than the ones we've talked about so far. Up until this point, the tilings we've seen have been such that they could be extended outwards toward infinity on a plane. The Circle Limit drawings instead seem to converge toward a circle. In fact, these are still plane tilings, but in different kind of geometry, namely hyperbolic geometry.

In Euclidean geometry, the parallel postulate roughly states: For any line $L$ and point $P$ not on $L$, there is exactly one line parallel to $L$ that passes through $P$. This matches our intuitive notion of parallel lines from our everyday experiences (which Euclidean geometry models). But it is not necessary for geometry to include the parallel postulate, and hyperbolic geometry is the consequence of modifying the postulate to allow multiple lines to be parallel to $L$ and pass through $P$.

Taking a look at both Circle Limit I and Circle Limit III, both images are based on the Poincaré Hyperbolic Disc. In this model of hyperbolic geometry, we work within a circular disk. Lines are represented as circular arcs that intersect the disc at right angles. Circle Limit III highlights these arcs in the white stripes running along the fish. Two hyperbolic lines are considered parallel as long as their arcs don't intersect. Under this definition, we can confirm that multiple parallel lines can run through the same point — consider the following annotated version of Circle Limit III.

The red and pink arcs exemplify lines of this hyperbolic space. Call the red line $L$ and the labeled point where the fish mouths meet $P$ as in the modified parallel postulate. Then, we see that there are indeed multiple lines parallel to $L$ and passing through $P$ by observing the three pink arcs passing through $P$ yet not intersecting $L$.

Interestingly, all the birds in Circle Limit I are the same size with respect to their hyperbolic geometry, and the same goes for the fish in Circle Limit III. This is because the notion of measurement in the Poincaré model is different from the notion of measurement in the Euclidean plane in such a way that equally spaced objects (from the hyperbolic perspective) are closer to each other (from our eyes' perspective) as you move toward the perimeter of the disc.

There are many other interesting and unintuitive facts that emerge in hyperbolic geometry which you can read more about here.

Constructing Tessellations

Want to create your own tessellation? One technique takes inspiration from Escher's Liberation. Start with a simple tessellation (like the geometric examples above), and make a small modification. Let's start with the square-based one.

And then let's cut out a part of one of the tiles.

In order to maintain the symmetry, we must then copy our modification over to the other tiles (making sure to apply the appropriate translations, reflections and rotations).

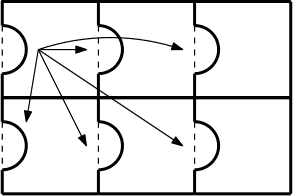
Repeat steps 2 and 3 until you are satisfied with the result.

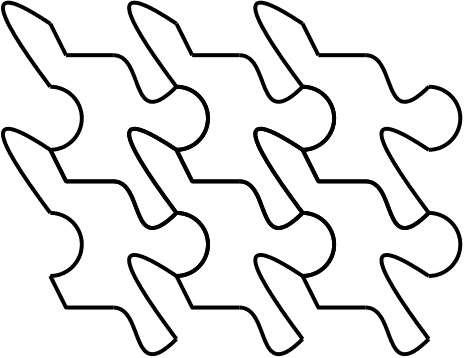
From this process, you can arrive at some interesting planar tessellations and gain more appreciation for Escher's art. If you're up for the challenge, you could try your hand at constructing a hyperbolic tiling in a similar way.